I should not have troubled you with my Algebraical Calculations but for your present inconvenient situation. I am however pleased to find your results, tho’ obtained a different way, the same with mine, having never that I remember turned to any Book on the Subject.—The rod used as a Pendulum does indeed require no correction, if it be no thicker than you propose, which will perhaps have weight sufficient.

I see nothing to Object to, in the four pages last received, except what I mentioned in my former letter, that you reduce 36.71428 French inches in 39.1923 English. Ferguson states the French foot equal to 12.788 inches English, that is 1/1000 part of an Inch less than Maskelines proportion, which I think most worthy of Credit. Therefore 36.71428 French inches cannot be more than 39.12824 English measure.

On the 5th. Article, “The practical difficulty resulting from the effect of the Machinery and moving power,” You have left a blank as if intending to say more. This is a subject which I have fully considered for several years past, and have written something on it which I intend to print. I have therein demonstrated that the force of the moving power may, at pleasure, be so applied to the pendulum as to make it vibrate either quicker or slower, in equal arches, than it would do in a Vacuum, at perfect liberty. And consequently that it may be so applied that it shall not change the times of Vibration at all. The Errors arising from unequal Arches of Vibration I have likewise proposed to remove with Geometrical accuracy, without using the Cycloid, and there is now going a Time Piece in our University so constructed.

The different elevations above the Surface of the Ocean, where the Experiments may be made, will scarcely merit Consideration.

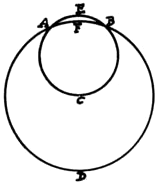
Let A, B, D be the general Surface of the Earth, and its Semidiameter [40]00 Miles. Let A. E. B. be an Elevated tract of Country [embraced?] by a Sphere passing thro’ the Center of the Earth, and [whose gr]eatest Elevation F. E. is 2. miles. Then will its Diameter A B be 246 miles, and a pendulum at E must be 1/4000 part longer than at A or B.

Some years ago I endeavored to determine the probable lenghth of a Degree of Longitude in any given Latitude, from the 9 Measures of different Degrees of Latitude given by Maskeline in the Transactions. I thought the fairest way would be to assume such a Magnitude for the Earth, and such a proportion between its Axis and Equatorial Diameter, as would make the Supposed Errors of Measurement the least possible, and likewise divide them equally between Excess and Defect. This I found will happen if a degree of Longitude at the Equator be 57169 French Toises and the polar to the Equatorial Diameter as 271½ to 272½. For then,

			Lenghth of a degree in F. Toises
			Calculated	Measured		Errors of Measurement
At the Equator			56750.00	56750	+	0.00
Latitude	33.°	18″	56938.57	57037	+	98.43
	39.	12	57000.09	56888	−	112.09
	43.	0	57041.40	56979	−	62.40
	44.	44	57060.38	57069	+	8.62
	45.	0	57063.33	57028	−	35.33
	47.	40	57092.60	57091	−	1.60
	49.	23	57111.33	57074	−	37.33
	66.	20	57277.30	57419	+	141.70
			Sum of Errors		+	248.75		Least possible if the figure of the Earth be a regular Spheroid
					−	248.75,

I was Surprized to find that I cou’d not assign any portion of the Errors to the Equator Measurement, without encreasing the Sum of all the Errors.

All this I mention to Shew the uncertainty we are in respecting the figure of the Earth, and consequently the lenghth of Pendulums in different Latitudes.

You will please to let me know if I am to return all the papers you have transmitted to me. I am, Dr Sir, with great respect, Your affectionate friend & Servant,