Note: this document has content that may require expanded/print view for best results (icons above right)

Enclosure: Thomas Jefferson’s Notes on Étienne Bézout’s Cours de Mathématiques, [before April 1815]

Enclosure

Notes on Étienne Bézout’s Cours de Mathématiques

[before Apr. 1815]

To reduce angles observed on an inclined plane to that of the horison. Bezout. trigonom. 320. cours d’Artillerie.

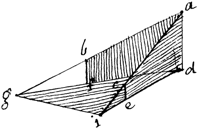
let d.g.i be the plane of the base of a hill, and a.b.c. points on it’s side at different heights.

		° ′
	suppose the ∠b.a.c. found by observation to be	62–37
	the ∠ of inclination b.a.d (a.d. being vertical)	88– 5
	the ∠ of inclination of c.a. to wit c.a.d.	78–17

it is required to find the ∠g.d.i. in the plane of the horizon.

let a.b. and a.c be prolonged until they meet the horizontal plane d.g.i. at g. & i.

consider a.d. as the radius of the tables,	then d.g. & d.i. become tangents of the tables,
	and a.g. & a.i. their secants; turn to the tables.

the ∠g.a.d.	= 88°–5′ has for it’s tabular	secant	29.90	neglecting subseqt fractions
	it’s	tangent	29.88
the ∠i.a.d.	= 78–17 has for it’s tabular	secant	4.92
		tangent	4.82

1st operation

in the △ a.g.i. given	a.g. = 29.9	required g.i
	a.i = 4.92
	∠a = 62°37′1
ag + ai : ag − ai :: ti + g/2 : ti − g/2 = t 49°–43′
then Si. ∠g : ai :: Si. ∠a. : gi = 28.

2d opern

in the right angled △ a.i.d.	given the ∠a.	= 78°–17′
	the hypoth. a.i	= 4.92. reqd d.i.
Rad. : 4.92 :: Si. 78°–17′ : d.i. = 4.8175

in the right angled △ a.g.d.	given the ∠a	= 88°–5′
	the hypoth. a.g	= 29.9. reqd d.g.
Rad : 29.9 :: Si. 88°–5′ : d.g. = 29.883

3d opern

in the △ g.d.i. given	d.g	= 29.883	required the ∠g.d.i.
	d.i	= 4.92
	g.i	= 28
dg : gi + di :: gi − di : gk − dk = 25.456
and di : Rad. :: kd : Si. ∠dik which is complement to the ∠gdi = 62°–39′–20″2

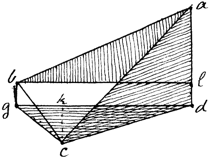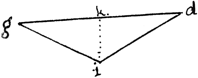
Suppose a the summit of a mountain: ad it’s height, b & c points at it’s foot, c being in the level of it’s base, or of the common horison, & b above that level.

				° ′ ″
	that the ∠	aCb	is observed to be	63–20–40
Required ad the height of mountn bg the height of station b.3 & the whole △ cdg, being the reduction of the points abc to the plane of the horizon.		aBc		66–39–20
		aCd		7–59–36
		aBl		7–48– 0
		bCg		0–28–39
	& that bc is by admeasuremt 6000. feet.

△ acd					° ′ ″
for ac.	Si. bAc	= 50°	: bc 6000	:: Si. aBc	= 66–39–20	: ac	= 7191.27
for cd	Rad.		: ac = 7191.27	:: S. cAd	= 82– 0–24	: cd	= 7121.4
for ad	Rad.		: ac.	:: S. aCd	= 7–59–36	: ad	= 1000.
△ abc
for ab	S. bAc	= 50°	: bc = 6000	:: S. aCb	= 63–20–40	: ab	= 7000.
△ abl
for bl	Rad.		: ab = 7000	:: S bAl	= 82–12– 0	: bl	= 6935.23

for al	Rad.		: ab.	:: S. aBl	= 7–48– 0	: al	= 950.
△ bcg
for cg	Rad.		: bc = 6000	:: S. cBg	= 89–31–21	: cg	= 5999.8
for bg	Rad.		: bc.	:: S. bCg	= 0–28–39	: bg	= 50.
△ cdg
	dg		: cd + cg	:: cd − cg	: dk − gk.
	6935.23		: 13121.2	:: 1121.6	: 2122.04
	then dk	= 4528.63 and gk = 2406.59
△ cdk						° ′ ″
for dCk	cd		: Rad.	:: dk.	: S. dCk	= 39–29–17
					then cDg	= 50–30–43

△ cGk
for gCk	cg		: Rad.	:: gk	: S. gCk	= 23–38–52
					then cGd	= 66–21– 8
		then the ∠dCg	= dCk + gCk			= 63– 8– 9
		then the ∠dCg

we have then the	height of the mountain ad = 1000.f
	height of the station b. = 50.f.

and the whole △ cdg, to wit		Note. the plane of the theodolite being adjusted to that of the horizon, will render truly (and without needing reduction) the horizontal angles at the two stations, whether they be in the same, or different horizontal planes; because, if different, as b. and c. their planes being parallel, the degrees on them will be coincident. but in this case the base measured on the inclined plane b.c. must be reduced to the horizontal one g.c. The above diagram supposes the plane of the theodolite adjusted to the inclined plane a.b.c. which renders necessary the reduction of it’s angles as well as lines to the horizontal plane d.g.c.
cd = 7121.4
cg = 5999.8
dg = bl = 6935.23
	° ′ ″
∠cDg =	50–30–43
∠cGd =	66–21– 8
∠dCg =	63– 8– 9

MS (MHi); filed with TJ’s Weather Memorandum Book, 1802–16; written entirely in TJ’s hand on both sides of a small sheet; undated, but composed prior to, and probably much earlier than, shipment of TJ’s library to Washington.

TJ based these notes in part on section 320 of Étienne Bézout, Cours de Mathématiques, a l’Usage du Corps Royal de l’Artillerie (Paris, 1788; Sowerby, description begins E. Millicent Sowerby, comp., Catalogue of the Library of Thomas Jefferson, 1952–59, 5 vols. description ends no. 3681), 1:334–6.

1. Equation interlined.

2. Page ends here.

3. Preceding six words interlined.

Index Entries

altitude; mathematical source for calculating search
Bézout, Étienne; Cours de Mathématiques, a l’Usage du Corps Royal de l’Artillerie search
books; on mathematics search
Cours de Mathématiques, a l’Usage du Corps Royal de l’Artillerie (É. Bézout) search
Jefferson, Thomas; Writings; Notes on Étienne Bézout’sCours de Mathématiques search
mathematics; books on search
mathematics; trigonometry search
scientific instruments; theodolites search
theodolite search

You Are Looking At

Source Project: Jefferson Papers
Title: Enclosure: Thomas Jefferson’s Notes on Étienne Bézout’s Cours de Mathématiques, [before April 1815]
Author: Jefferson, Thomas
Date: 13 October 1815

Reference

Cite as: “Enclosure: Thomas Jefferson’s Notes on Étienne Bézout’s Cours de Mathématiques, [before April 1815],” Founders Online, National Archives, https://founders.archives.gov/documents/Jefferson/03-09-02-0064-0002. [Original source: The Papers of Thomas Jefferson, Retirement Series, vol. 9, September 1815 to April 1816, ed. J. Jefferson Looney. Princeton: Princeton University Press, 2012, pp. 80–82.]